by Philip Pilkington
Article of the Week from Fixing the Economists
All too often a debate, or what sometimes passes for one, is due to one side not knowing the definition of terms. There are many reasons for this — sometimes, for example, people are just ignorant — but one reason that is often the case is that the use of a term that is semantically very general becomes congealed in a single usage.
This perversion of language was noted by George Orwell in his seminal essay Politics and the English Language. Orwell called this linguistic error a ‘dying metaphor’. In his essay he explains this error as such:
A newly invented metaphor assists thought by evoking a visual image, while on the other hand a metaphor which is technically “dead” (e.g. iron resolution) has in effect reverted to being an ordinary word and can generally be used without loss of vividness. But in between these two classes there is a huge dump of worn-out metaphors which have lost all evocative power and are merely used because they save people the trouble of inventing phrases for themselves. Examples are: Ring the changes on, take up the cudgel for, toe the line, ride roughshod over, stand shoulder to shoulder with, play into the hands of, no axe to grind, grist to the mill, fishing in troubled waters, on the order of the day, Achilles’ heel, swan song, hotbed. Many of these are used without knowledge of their meaning (what is a “rift,” for instance?). (Emphasis Original)
Economics (indeed, all social science, as Orwell hints at in the essay) is particularly prone to the abuse of dying metaphors. This is due to the nature of the discourse. Economics, for example, often uses mathematical or geometrical metaphors and because of the seemingly concrete form that they take it is easy for the non-thinking student who learns by rote to ‘reify‘ the metaphors and mistake them for the actual relationships they are describing; the linguistic counterpart of this error is that the mathematical or geometrical metaphor ‘dies’, as Orwell put it.
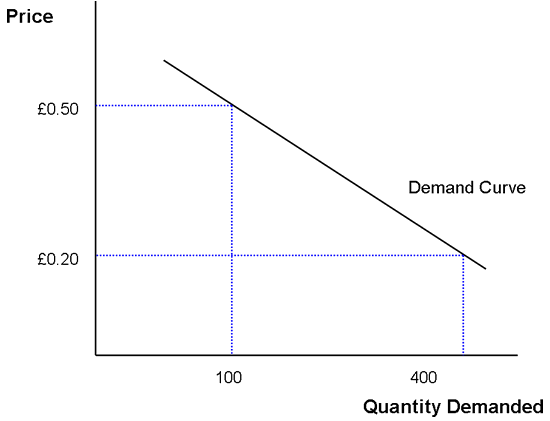
A good example of this is the downward-sloping demand curve. What is a downward-sloping demand curve? Well, it is, as are all linguistic terms, exactly what it says that it is: it is a demand curve that slopes downwards.
The best known of such demand curves is, of course, the one represented in the classic supply and demand graph that first year undergraduates are taught and which can be seen below.
This curve, when applied in basic microeconomics, depicts a number of relationships (diminishing marginal utility, substitution effects etc.) that do not here concern us. More important is that the curve is said to represent the Law of Demand. The Law of Demand can be summarised by the mathematical relationship:
What that means is that quantity demanded is a function of price and this is understood to be an inverse relationship. These are really the only true mathematical properties of the above curve. The economic rationale for why these relationships hold is completely separate from the mathematical metaphor being used to describe them.
The downward-sloping demand curve in this regard is the metaphor; it is not the relationships that the metaphor seeks to describe. This is how all metaphors function. If I say that a man is boiling under the surface like a pressure cooker I mean that he is extremely angry and that this anger is likely to be released at some point. The metaphor — in this case, the boiling pressure cooker — is not identical with the relationship it seeks to explain — in this case, the uncontainable anger. For this reason the metaphor can be transferred. For example, if I said that a lover was boiling under the surface like a pressure cooker. The metaphor remains the same, but the underlying relationship it seeks to describe is almost entirely the opposite of the previous one.
Now, that’s all fine and dandy but why does this lead to confusion in economic discourse? Simple. Because some people begin to mistake the mathematical or geometrical metaphors for the relationships they describe. Thus a downward-sloping demand curve becomes equated in the minds of unthinking and unreflective people as being wholly synonymous with, for example, the relationships that underlie the microeconomic demand curve taught in undergraduate classes (i.e. diminishing marginal utility, substitution effects etc.).
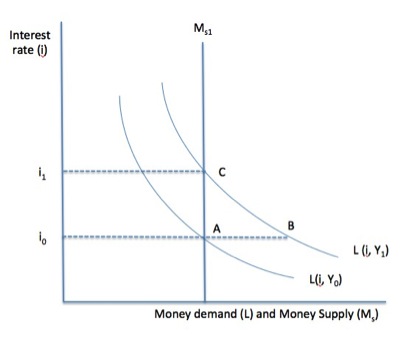
Far be it from being a simple example of poor thinking and poor language use, this confusion can cause all sorts of confusions. You see, we find downward-sloping demand curves all over the place in (marginalist) economics. Take, for example, the money demand curve (L) in the ISLM model as laid out below:
Is this a demand curve? Certainly. Is it downward-sloping? Evidently so. Does this make it a ‘downward-sloping demand curve’? Of course! But are the relationships that it describes the same as those underlying the microeconomic demand curve previously discussed? Absolutely not. But again, the mathematical or geometric metaphor should not be confused with the relationships it describes — just like the boiling pressure cooker should not be confused with the uncontainable anger; to do so is a logical fallacy.
Let us bring this further still, because I think there is some insight into economics in the above discussion. Take again our Law of Demand as expressed in algebraic form:
Does the money demand curve in the above graph conform to this? Yes, it does. In the graphical representation it can clearly be seen that the quantity of money demand is inversely related to the interest rate which is, of course, the price of money. Thus, the conception of money in the ISLM framework can be said to depend not just on a downward-sloping demand curve for money (this is self-evident) but also on the Law of Demand.
Here is a hypothesis I want to lay in front of the reader: marginalist economics rests on its ability to sneak the Law of Demand, in a variety of different and sometimes unrecognisable forms, into many, many economic relationships. Indeed, this is perhaps one of the key pillars of the entire discourse.
With that considered, let us once again reinforce our original point: economists and mathematicians, often not being the most critical thinkers, have a terrible tendency to confuse their metaphorical representations with the relationships that underlie said metaphorical representations. This can lead to all sorts of embarrassing confusions — confusions that can, on occasion, be very destructive and stifle thinking altogether. If economists can train themselves to avoid the pitfall of dying metaphors, their ability to think about economic arguments and relationships and to present these to themselves and others will be greatly improved.
Update: There has been much discussion on various Facebook pages about the so-called Law of Demand in light of this post. I should point out that I was using it both as a point of departure and as a means to point out an inherent structural feature of marginalist economics. I do not in any way conceive it to be an actual “law” and think that its name is just another instance of the extreme pseudo-scientific pretentiousness of marginalist economics. For a very compact, dense and pointed overview of its actual epistemological status see the following post by Lord Keynes.